Paradoxes – Why Achilles loses the tortoise race

This sentence is not true. Right? If the statement is true, it states that it is not true. But if it is not true, then that would mean that the statement is actually true… If you are already confused, you might be ready to start diving deeper into the weird and fascinating questions of logic and philosophy.

Paradoxes are statements that seem to contradict themselves. Like the first sentence of the article, they seem to be true but at the same time cannot be true. They do not seem to be logical at all. But this is exactly the reason why philosophers and logicians in general are very interested in Paradoxes. As Paradoxes question our perception of our world and language, they can cause the invention of surprising new theories and enhance our knowledge of the world. But paradoxes are not as abstract as they now might seem to you. As you will see while reading this article, they can suddenly appear in the world around you but affect everything that you believed the reality to be.

The first example I want to explain was invented by Zeno of Elea, a Greek philosopher who lived in the 5th century BC. He was convinced of the fact that motion is nothing but an illusion. You may walk, run or fly, but you would never really move. At first, this might seem to be a rather awkward thought as we are all used to the idea of motion, but Zeno’s arguments will teach us a philosophical lesson. His paradoxes play with our idea of infinity.

Imagine watching an unusual race of unusual participants in Ancient Greece: Achilles, the famous warrior competes against a tortoise. The tortoise is allowed to start with a lead of, let’s say 100 meters. 3, 2, 1… the race begins! Who do you bet on?

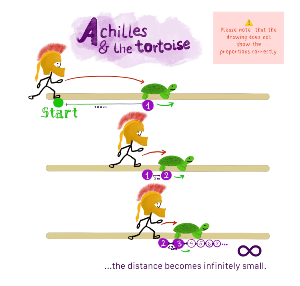
Achilles will never reach the tortoise although he is faster. But why? After 10 seconds, Achilles ran 100 meters. At the same time, the tortoise manages to move only a few meters. So, Achilles has reached the point (1) where the tortoise started and now is still a few meters away from it. But when he reaches point (2) the tortoise reached after 10 seconds, the tortoise is already gone and reached a third point. This is because it is moving at a constant speed. When he then reaches point (3) the tortoise has moved further. Maybe you need an illustration to imagine it. This continues for infinity – and the tortoise is always ahead of Achilles. But when you think of it again, this is absurd. A fast and trained runner who is faster than the tortoise must in every case overtake it.

That was the aim of Zeno’s argumentation: To show that the idea of motion can lead to absurd conclusions, and therefore is false.

Another paradox that shows the difficulty of motion is the paradox of the arrow. But you can imagine anything that moves e.g., a flying bird or a rocket. Imagine looking at it for an instant of time as if you would take a photo. At this moment, it is not moving – as there is no time going by it cannot move further. This is the same for every other instant of time. In conclusion, at every instant of time, motion does not exist – so it does not exist at all!

But solutions to these paradoxes have already been found. You may now want to try to disprove Zeno’s arguments by yourself. You can take your time to think about it and continue reading later.

The solution to the first paradox can be solved mathematically. Perhaps you have already intuitively spotted the mistake in Zeno’s argument: It is possible indeed to divide any distance into infinite parts. But you cannot conclude that everything is therefore infinite. If you cut a cake into two pieces, four pieces, eight pieces, and so on, you could theoretically continue to do so for infinity. But this does not change the fact that there is only one cake. Cutting the cake infinitely does not make it impossible to eat the cake. So Achilles does not have to run forever, he does not need infinity to overtake the tortoise. From a mathematical point of view, series or infinite sums can have finite results. But it was not until the 17th century that this mathematical problem was solved using the concept of limits.
Many philosophers have studied the second paradox. The simplest answer to this argument is that the concept of motion requires the concept of time. So motion is defined as a change in position over time, and Zeno’s argument makes no sense. Besides, it is also possible to solve this paradox by using limits again.

There are an uncountable number of paradoxes. Some deal with infinity, such as Zeno’s paradoxes. Some deal with self-reference, identity, statistics, linguistics or even quantum mechanics, such as the paradox of Schrödinger’s cat.
For example, the Cretan Epimenides of Knossos once said: “All Cretans are liars”. This self-referential paradox from the 6th century BC is one of the most famous paradoxes. Its character is similar to the first sentence of this article.
The Ship of Theseus is another paradox – but it is about personal identity. The ship of the mythical hero Theseus was admired and honoured by the Athenians in an annual ceremony. This went on for centuries – and gradually every single part of the ship was broken and then replaced. Is it still the same ship, still the ship of Theseus?

One final paradox: If you know what you are searching for, there is no need to ask. But if you do not know what you are looking for, how can you ask for it? It is impossible.
This paradox, also known as Meno’s paradox, can also be solved. However, transferring it to the question of epistemology makes it rather interesting. How can human beings acquire knowledge? Are we already born with all knowledge? Do you first have to make experiences in order to know something? A whole area of philosophy deals with this problem.

To put it in a nutshell: Paradoxes change the way we think about the world. They can confuse us – but at the same time we can learn something new. As Plato, the famous Greek philosopher, once wrote in his dialogue Theaetetus: Philosophy begins in wonder.

References: